29 The Reynolds number

Now that we have a mathematical representation for friction in a fluid, let’s add it to the Navier-Stokes equation. We will only write the x-component momentum equation below.  But, we are considering the diffusion of eastward momentum (u) in each of the three directions, so the friction term appears on the right as \nu \nabla^2 u.

    \[ \frac{Du}{Dt}=\frac{\partial u}{\partial t}+u \frac{\partial u}{\partial x} +v \frac{\partial u}{\partial y} +w \frac{\partial u}{\partial z}=\nu \nabla^2 u+\sum F^x \]

In the second step, we have expanded the Lagrangian acceleration into its several terms. The first term is the Eulerian acceleration.  The next three terms, combined, are the divergence of the advective flux of eastward momentum, also called the inertial terms. It is the inertial terms that make the momentum equation nonlinear and lead to turbulence.

In a given physical situation, we can make a scaling argument to assess how large these terms are likely to be, relative to other terms in the equation. Let’s compare the inertial terms to the viscous term, for example. We know from observing a given situation something about the speed of a current and how quickly it changes in space. We assume rough orders of magnitude, or scales, for the variables in these terms:

L = the length scale of the flow, i.e., the distance over which we observe the current change from zero speed to its typical velocity, and

U = the velocity scale, or speed of this current

We also know \nu, the viscosity (i.e., the diffusivity for momentum), as a physical property of the fluid that we could measure.

Let’s assume for now that there is no particular orientation of the current, so that each of the three inertial terms could have an order of magnitude that we could estimate as

    \[ u \frac{\partial u}{\partial x}, v \frac{\partial u}{\partial y}, w \frac{\partial u}{\partial z} \sim U\frac{U}{L} \]

where we use the “ \sim “ operator to indicate “roughly equal in size.” Similarly, the viscous terms could be estimated as

    \[ \nu \frac{\partial^2 u}{\partial x^2},\nu \frac{\partial^2 u}{\partial y^2},\nu \frac{\partial^2 u}{\partial z^2} \sim \nu \frac{U}{L^2} \]

The Reynolds number, Re, is the ratio of the estimated sizes of the inertial to viscous terms using these scaling constants:

    \[ Re = \frac{U\frac{U}{L}}{\nu \frac{U}{L^2}} = \frac{UL}{\nu} \]

It is one of several important nondimensional numbers used to assess the relative sizes of terms in the momentum equation.

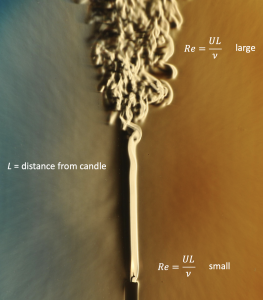
When Re is large, the inertial terms are much more important than the viscous terms and the fluid is turbulent.  When Re is small, friction dominates the system, which then has linear dynamics and smooth flow patterns – laminar flow.

The Reynolds number increases as L, the distance from the candle, increases. The flow becomes turbulent at large values of the Reynolds number.

 

Media Attributions

License

Icon for the Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License

Physics Across Oceanography: Fluid Mechanics and Waves Copyright © 2020 by Susan Hautala is licensed under a Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License, except where otherwise noted.

Share This Book