19 The Pressure Gradient Force (PGF)

 

Illustration of the x-component of the pressure gradient force (PGF) associated with an eastward decrease in sea level across a control volume. y- and z- components are not shown.

If the pressure varies in space within the fluid (i.e., it has a nonzero gradient), then there will be a net force on fluid parcels called the pressure gradient force. It is a force per unit mass and thus has units of Newtons per kg. (Recall that 1 Newton is the force required to accelerate an object with a mass of 1 kg at 1 m s-2.)

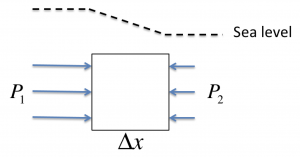
In the diagram, the pressure is decreasing to the right (as we move toward positive x). The pressure gradient would be directed to the left, toward higher pressure.

Recall that pressure is directed inward on all sides of a fluid parcel. There is a greater magnitude force, Pressure \times Area, directed toward positive x on the left, than directed toward negative x on the right. The left and right sidewalls both have Area=\Delta z \hspace{2pt} \Delta y. If we give the right face subscript “2” and the left face subscript “1”, we can write the net force in the x-direction as

    \[ (P_1-P_2)\Delta z \hspace{2pt} \Delta y. \]

The PGF is the net force per unit mass (Mass = Density \times Volume), so using the Greek symbol \rho, “rho”, for the density, we get

    \[ PGF = \frac{Net force}{Mass}=\frac{(P_1-P_2)\Delta z \hspace{2pt} \Delta y}{\rho \Delta x \hspace{2pt} \Delta z \hspace{2pt} \Delta y }=\frac{1}{\rho}\frac{(P_1-P_2)}{ \Delta x}=-\frac{1}{\rho}\frac{\Delta P}{ \Delta x}, \]

In the last step above, the sign is changed because we want to take the differences, the \Delta‘s, in the same order, i.e., \Delta P = P_2-P_1 and \Delta x = x_2 - x_1. If we now use the partial differential notation, making these differences infinitesimally small, we get

    \[ PGF = -\frac{1}{\rho}\frac{\partial P}{ \partial x}, \]

This is only the x-component of the pressure gradient force, and so we also need to consider differences in the other coordinate directions.

 

Key Takeaways

The pressure gradient force is defined as:

    \[ PGF=\underline{\textbf{F}}_P=-\frac{1}{\rho}\nabla P=-\frac{1}{\rho} \left( \frac{\partial P}{ \partial x},\frac{\partial P}{ \partial y}, \frac{\partial P}{ \partial z} \right) \]

where P is pressure and \rho is the fluid density.  It is a force per unit mass, with units N kg^{-1}, equivalent to m s^{-2}.

The PGF has the opposite sign to the pressure gradient.  It is directed from high to low values of pressure, i.e., it is a down-gradient vector.

Note: In the expression above, we used vector \underline{\textbf{F}} to represent force per unit mass. Before we have used it to represent flux. The context should make it clear which physical quantity we are talking about, and this is a very common convention for representing both kinds of fields.

Media Attributions

License

Icon for the Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License

Physics Across Oceanography: Fluid Mechanics and Waves Copyright © 2020 by Susan Hautala is licensed under a Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License, except where otherwise noted.

Share This Book