57 The Doppler shift

Some nice animations and discussion of the Doppler effect can be found on Dan Russell’s webpage The Doppler Effect and Sonic Booms. Here we will develop this idea mathematically.

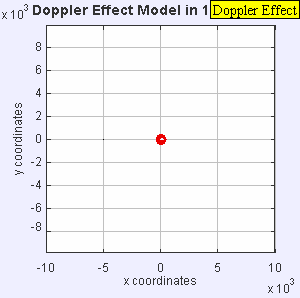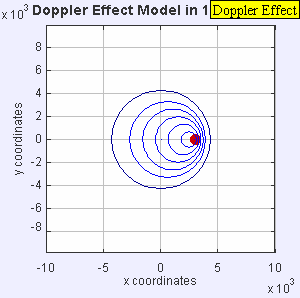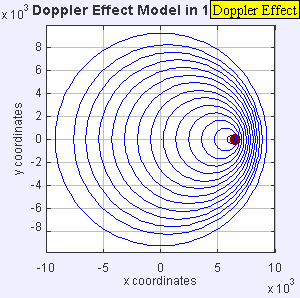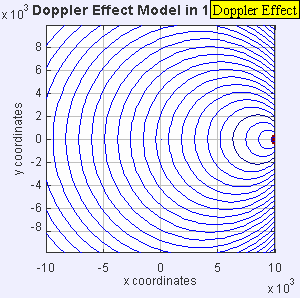
Consider the sound source moving toward positive x, with a positive velocity, u, as shown in the figure to the left. Notice that, for a nonmoving observer, the wavelength (the distance between crests) is smaller in front of the moving source. This is because by the time one crest has moved some distance towards positive x at the wave speed (given by the medium), the source has moved some distance in the same direction. The next wave crest is thus created with a shorter separation than if the source were not moving.

Similarly, the wavelength behind the moving source is larger.

We develop expressions for the associated shift in frequency corresponding to these wavelength differences (called the Doppler shift) as follows. We will use the subscript “0” to indicate properties of a sound wave from a stationary source, often called the intrinsic properties. We will use subscripts “F” and “B” to indicate the properties of the Doppler-shifted wave as observed by a stationary observer located, respectively, in Front and Behind the moving source. Once a wave crest is emitted, it propagates at the speed given by the medium such that

Over a wave period, the sound source moves a distance . Thus the wavelength in front of the wave is reduced from its intrinsic value by this distance,

The wavenumber in front of the wave is thus

The frequency in front of the wave is

We use similar arguments behind the moving observer.

For small velocity, relative to the sound speed, these equations can be applied similarly to the case of a moving observer and a stationary source – it is only the velocity difference that matters.